Industrial Geometry

Industrial Geometry — an emerging research area

Industrial geometry is based on computational techniques which originated in various areas of applied geometry. To give a few examples, the methods of Computer Aided Geometric Design form the mathematical foundation of the powerful CAD technologies available today. Computer Vision provides methods for inspecting and analyzing images and videos. Image processing is used to reconstruct geometric features from digital image data, such as X-ray or computer tomography images. Computational Geometry provides efficient algorithms for solving fundamental geometrical problems. Robot kinematics deals with geometric problems which occur in connection with robot mechanical systems.

In recent years, these different areas of research have started to become increasingly interconnected, and begun even to merge. A driving force in this process is the increasing complexity of applications, where one field of research alone would be insufficient to achieve useful results. Novel technologies for acquisition and processing of data lead to new and increasingly challenging problems, whose solution requires the combination of techniques from different branches of applied geometry.

Objectives, long-term benefits and future perspectives

The main goals of the NRN are:

• Initializing a close cooperation between Austrian research groups working in areas which are important for Industrial Geometry.
• Building up an internationally leading research team in Industrial geometry by doing joint research on the many projects which have been identified by the members of our team.
• Development of new methods for the solution of important Industrial geometry problems.
• Developing new university course material in central areas of Industrial geometry, thereby introducing young researchers to Industrial geometry, which is necessary for growth and continuing excellence of this research area.
• Creating an international scientific community for Industrial Geometry.
• Defining and emphasizing new directions for geometry education in Austria, which draws from a long tradition of constructive geometry.
• Strengthening Industrial Mathematics as an already existing area of excellence in the Austrian research landscape.

Integration of the subprojects into the overall concept

One of the main goals of the proposed NRN is the initialization of a close cooperation between different research groups working in various areas of Industrial Geometry. The structure of the present proposal reflects this by showing the close interrelation of the research problems we intend to study. The fact that the majority of such problems has its origin in one of those areas and has been formulated within the related theoretical background does not disagree with this viewpoint.

In view of the wide range of different topics involved, ranging from classical geometry to robotics and computational geometry, the combined knowledge of all groups participating in this NRN will be needed for its successful implementation. Specific relations and expected synergies between the sub-projects are described in detail within each subproject. Please refer to project pages for more details.

Events

Events 2011

"8th European Research Week on Geometric Graphs and Pseudo-Triangulations (formerly PT-week)": The workshop will take place from Monday to Friday, November 7 – 11, 2011, near Graz.
Closing Event of the National Research Network 'Industrial Geometry' from Wednesday to Friday,
October 19 – 21, 2011, in Vienna, Austria. Each half day of the workshop will be devoted to a special topic, e.g. Computational Geometry, variational methods, ...
Geometry Workshop Obergurgl 2011 from Sunday June 19 to Thursday June 23, 2011.

Events 2010

7th NRN seminar: The 7th NRN seminar took place at Strobl / Wolfgangsee, Austria from Wednesday to Friday, October 27 - 29, 2010. The program and the presentations are now available.
International NRN Workshop 2010 in Obergurgl from Wednesday to Friday, April 7 - 9, 2010.

Events 2009

"6th European Pseudo-Triangulation Research Week": The workshop took place from September 28 - October 2, 2008, near Graz.
International NRN Workshop "Surfaces, Meshes, Geometric Structures" in Admont: The conference will take place at Roethelstein castle, overlooking the city and monastery of Admont, from Monday to Thursday, July 6 - 9, 2009. Topics of this meeting include: polyhedral surfaces and discrete differential geometry in general; computational geometry of mesh- and point-based data; hinge mechanisms and tensegrities; questions of statics, rigidity and flexibility; geometric optimization. In particular we are interested in applications.
6th NRN seminar: The 6th NRN seminar took place at Strobl / Wolfgangsee, Austria from Wednesday to Friday, April 15 - 17, 2009. The program and the presentations are now available.

Events 2008

NRN workshop "Fifth European Pseudo-Triangulation Research Week": The European Pseudo-Triangulation Research Week came back to where it was born in 2004. It took place from October 6th to 10th 2008 near Graz. The group of local researchers was completed by international participants: Michael Hoffmann (ETH Zürich), Elena Mumford (TU Eindhoven), Pedro Ramos (Universidad de Alcalá), Günter Rote (FU Berlin), and Bettina Speckmann (TU Eindhoven).
5th FSP seminar: The 5th FSP seminar took place at Strobl / Wolfgangsee, Austria from Wednesday to Friday, March 26 - 28, 2008. The program is now available here.

Events 2007

Workshop on Computational Methods for Algebraic Spline Surfaces , September 10 - 14, 2007, in Strobl / Wolfgangsee.
Workshop on Polyhedral Surfaces and Industrial Applications: September 15 - 18, 2007, Strobl / Wolfgangsee.
4th FSP seminar,. The 4th FSP seminar took place at Schloss St.Martin, Graz, Styria, Austria from Thursday to Friday, March 22 - 23, 2007. For details click here, (program in PDF-format).
23rd European Workshop on Computational Geometry: FSP-related event: The 23rd European Workshop on Computational Geometry took place at Graz University of Technology, Graz, Styria, Austria from Monday to Wednesday, March 19 - 21, 2007.

Events 2006

FSP workshop at Vorau, Steiermark, Austria: From November 27-29, 2006 a FSP seminar (program in PDF-format) took place at Stift-Vorau, Styria, Austria.
3rd FSP seminar on "Variational, PDE, and Level Set Methods": Within the FSP program "Industrial Geometry" founded by the Austrian Science Foundation (FWF), a workshop on "Variational, PDE, and Level Set Methods" was held in Obergurgl, Tyrol, Austria (September 1 - 5, 2006).
2nd FSP seminar: 2nd FSP seminar took place at Bifeb Strobl / Wolfgangsee, Austria from Monday to Thursday, June 19 - 22, 2006, here is the program (in PDF-Format), and here you find the schedule with download-possibilities.

Events 2005

1st FSP seminar Including educational- and CGAL-workshops took place at Schloss St.Martin, Graz, Styria, Austria from Wednesday to Friday, November 23 - 25, 2005. For details click here.

Publications

The following list contains all publications within this NRN separated by subprojects. Most of the publications can be found as preprints in the NRN report series.

• Project S09201 Coordination and Service: Publications (the educational/scientific part of subproject 1 was discontinued after the 1st funding period)
• Project S09202 Coupling evolving level sets with curves and surfaces: Publications
• Project S09203 Constrained Level Sets and Shape Understanding: Publications
• Project S09205 Computational Geometry: Publications
• Project S09206 Applications of Higher Geometries: Publications
• Project S09207 Pattern and 3D Shape Recognition: Publications (only first funding period, subsequently merged with subproject 3)
• Project S09209 Computational Differential Geometry (only second funding period)

Publications, subproject 07

Mongraph

15. Scherzer, M. Grasmair, H. Grossauer, M. Haltmeier, and F. Lenzen. Variational Methods in Imaging. Springer, 2007. submitted.

Journal articles

13. Grasmair and A. Obereder. Generalizations of the taut string method. Numerical Functional Analysis and Optimization 29/3 (2008), 346-361.
14. Fidler, M. Grasmair, and O. Scherzer. Identifiability and reconstruction of shapes from integral invariants. Inverse Problems and Imaging 2/3 (2008), 341-354.
15. Fuchs, B. Jüttler, O. Scherzer, and H. Yang. Combined evolution of level sets and B-spline curves for imaging. Computing and Visualization in Science (2008), to appear.
16. Fuchs and O. Scherzer. Regularized reconstruction of shapes with statistical a priori knowledge. International Journal of Computer Vision 79/2 (2008), 119-135.
17. Burger, K. Frick, S. Osher, and O. Scherzer. Inverse total variation flow. Multiscale Model. Simul. 6/2 (2007), 366-395.
18. Fidler, M. Grasmair, H. Pottmann, and O. Scherzer. Inverse problems of integral invariants and shape signatures. (2007), submitted.
19. Hofmann, B. Kaltenbacher, C. Pöschl, and O. Scherzer. A convergence rates result for Tikhonov regularization in Banach spaces with non-smooth operators. Inverse Problems 23/3 (2007), 987-1010.
20. Frühauf and H. Grossauer. Solving constraint ill-posed problems using ginzburg-landau regularization functionals. Journal of Inverse and Ill-posed Problems (2007), accepted.
21. Haltmeier, R. Kowar, A. Leitao, and O. Scherzer. Kaczmarz methods for regularizing nonlinear ill-posed equations II: Applications. Inverse Problems and Imaging 1 (2007), 507-523.
22. Haltmeier, A. Leitao, and O. Scherzer. Kaczmarz methods for regularizing nonlinear ill-posed equations I: Convergence analysis. Inverse Problems and Imaging 1 (2007), 289-298.
23. Hofmann, Kaltenbacher, C. Pöschl, and O. Scherzer. A convergence rates result in banach spaces with non-smooth operators. Inverse Problems 23 (2007), 987-1010.
24. Obereder, O. Scherzer, and A. Kovac. Bivariate density estimation using BV regularisation. Computational Statistics and Data Analysis (2007), to appear.
25. Resmerita and O. Scherzer. Error estimates for non-quadratic regularization and the relation to enhancing. Inverse Problems 22 (2006), 801-814.
26. Lenzen, O. Scherzer, and S. Schindler. Robust reconstruction from chopped and nodded images. Astronomy & Astrophysics 443 (2005), 1087- 1093.
27. Scherzer, W. Yin, and S. Osher. Slope and G-Set Characterization of Set-Valued Functions and Applications to Non-Differentiable Optimization Problems. Comm. Math. Sci. 3 (2005), 479-492.

Refereed articles in books and conference proceedings

3. Pöschl and O. Scherzer. Characterization of minimizers of convex regularization functionals. In AMS-SIAM Special Session on Frames and Operator Theory in Analysis and Signal Processing, number 451 in Contemporary Mathematics, pages 219-248, 2008.
4. Abhau, W. Hinterberger, and O. Scherzer. Segmenting surfaces of arbitrary topology: A two-step approach. In S. Y. Emelianov and S. A. McAleavey, editors, Medical Imaging 2007: Ultrasonic Imaging and Signal Processing, volume 6513 of Proceedings of SPIE, San Diego, CA, 2007.
5. Frick and O. Scherzer. Applications of non-convex BV regularization for image segmentation. In X.-C. Tai, K.-A. Lie, T. F. Chan, and S. Osher, editors, Image Processing Based on Partial Differential Equations, Mathematics and Visualization, pages 211-228, New York, 2007. Springer Verlag.
6. Frick and O. Scherzer. Convex inverse scale spaces. (2007), 313-325, Lecture Notes in Computer Science 4485.

Technical Reports

13. Grasmaier. Convex regularization methods with non-uniform total variation penalization. Technical Report 57, FSP 092: Joint Research Program of Industrial Geometry, October 2007.
14. Colutto, F. Frühauf, M. Fuchs, and O. Scherzer. The CMA-ES on Riemannian manifolds to reconstruct shapes in 3D voxel images. Technical Report 70, FSP 092: Joint Research Program of Industrial Geometry, May 2008.
15. Fidler, M. Grasmair, H. Pottmann, and O. Scherzer. Inverse problems of integral invariants and shape signatures. Technical report, FSP report no. 40, 2007.
16. Fuchs and O. Scherzer. Segmentation of biologic image data with a-priori knowledge. Technical report, FSP report no. 52, 2007.

(This subproject was merged with subproject 3 after the first funding period)

La pleine lune

La pleine lune est la phase lunaire la plus spectaculaire lorsque toute la face de la lune est éclairée.

Quand est-ce la pleine lune?

100% éclairé : À la pleine lune, toute la face de la lune est illuminée par les rayons du soleil et elle peut être suffisamment lumineuse pour éclairer des nuits autrement sombres.

Techniquement, cette phase primaire de la Lune ne dure qu'un instant, l'instant où le Soleil et la Lune sont alignés de part et d'autre de la Terre (voir illustration). Donc, l'heure exacte de la pleine lune est pendant la journée sur certaines parties de la planète.

Cependant, la Lune peut sembler pleine un jour avant ou après alors que plus de 98% du disque de la Lune est illuminé.

Par conséquent, il peut être difficile de faire la différence entre une Pleine Lune et le dernier stade d'une Lune Gibbeuse Cire ou le début d'une Lune Gibbeuse Décroissante .

Terre au milieu

La position de la Lune dans l'espace à la pleine lune.
Le terme technique pour l'alignement de la pleine lune est syzygie du système Soleil-Terre-Lune.

Lorsque le côté de la Lune que nous pouvons voir de la Terre est entièrement éclairé à la pleine lune, le côté opposé est dans l'obscurité, et vice versa à la nouvelle lune .

La Pleine Lune est visible dans le ciel approximativement du coucher au lever du soleil . Au moment précis de l'alignement de la pleine lune, la lune n'est visible que dans la partie nocturne de la Terre, à quelques exceptions près.

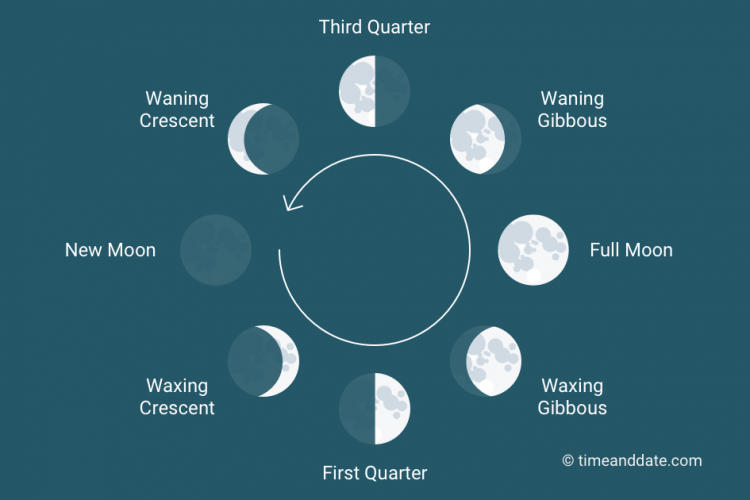
Une phase de lune primaire

La pleine lune est la troisième des quatre phases primaires de la lune qui se produisent à des moments précis dans le temps. Les trois autres sont la Nouvelle Lune , le Premier Quart de Lune et le Troisième Quart de Lune .

De plus, il existe quatre phases intermédiaires qui prennent le temps entre les phases primaires. Celles-ci sont appelées Waxing Crescent Moon , Waxing Gibbous Moon , Waning Gibbous Moon et Waning Crescent Moon .

Orbite elliptique

La Lune orbite autour de la Terre dans le sens antihoraire sur un chemin elliptique, et le même côté de la Lune fait toujours face à la Terre. Cependant, au fil du temps, la Lune bascule légèrement du nord au sud et vacille un peu d'est en ouest. Ce mouvement, connu sous le nom de libration lunaire , permet de voir jusqu'à 58% de la surface de la Lune depuis la Terre, bien que seulement 50% à la fois.

Supermoon et Micromoon

Le point de l'orbite de la Lune le plus proche de la Terre est appelé périgée et le point le plus éloigné est appelé apogée . Lorsque la pleine lune se rapproche de périgée, il est connu comme un Supermoon ou Super Full Moon.
Lorsqu'une Pleine Lune est proche de l' apogée , elle s'appelle Micromoon et il est possible d'observer la lune avec un téléscope.

Marées supérieures à la pleine lune
La plus grande différence entre la marée haute et la marée basse se situe autour de la pleine lune et de la nouvelle lune . Au cours de ces phases de la Lune, les forces gravitationnelles de la Lune et du Soleil se combinent pour tirer l'eau de l'océan dans la même direction. Ces marées sont appelées marées de printemps ou marées royales.

Provoque des éclipses lunaires

Environ 2 ou 3 fois par an , la Pleine Lune se rapproche des nœuds lunaires. Ce sont les points où l'orbite de la Lune traverse l'écliptique, qui est la trajectoire du Soleil, vue de la Terre. Lorsque cela se produit, la Terre projette son ombre sur la Pleine Lune, provoquant une éclipse lunaire .

Les éclipses solaires , en revanche, ne peuvent se produire que si la Lune s'approche des nœuds lunaires autour de la Nouvelle Lune.

Blue Moon est une pleine lune

La plupart des années ont 12 pleines lunes, 1 chaque mois. Cependant, notre calendrier n'est pas parfaitement synchronisé avec les événements astronomiques. Par conséquent, de temps en temps, une année a 13 pleines lunes. Lorsque cela se produit, au moins une de ces pleines lunes est appelée Blue Moon .

Pleine lune dans les calendriers

Symbole de calendrier pour la pleine lune
Le symbole de la pleine lune dans les calendriers modernes est un cercle complètement blanc.

Les autres symboles de phase lunaire principaux dans les calendriers sont:
Symbole de calendrier pour la nouvelle lune= Nouvelle Lune, Symbole de calendrier pour le premier quart de lune= Premier trimestre, Symbole de calendrier pour le troisième quart de lune= Troisième trimestre

Calendrier avec phases de lune

Affecte les marées
Les marées sur Terre sont principalement générées par l'attraction gravitationnelle de la Lune d'un côté de la Terre à l'autre. La gravité de la Lune peut provoquer de petits flux et reflux dans les continents appelés marées terrestres ou marées terrestres solides. Celles-ci sont plus importantes pendant les pleines et les nouvelles lunes parce que le soleil et la lune sont alignés sur les mêmes côtés ou les côtés opposés de la Terre.

La pleine lune dans la culture
La Lune a influencé la culture humaine pendant des millénaires, et la phase de la pleine lune en particulier. La date du dimanche de Pâques , par exemple, est déterminée en fonction de la pleine lune et de l' équinoxe vernal .

La Lune a également inspiré l'invention d'innombrables divinités, comme la déesse romaine Luna ou son homologue nordique Máni, qui a donné son nom à lundi . Et, même aujourd'hui, les gens utilisent d'anciens noms de la pleine lune , comme Harvest Moon et Strawberry Moon .

Sorcières et loups-garous
Dans le passé, il était courant de penser que de nombreuses formes de maladie mentale étaient causées par la Lune, d'où le nom de fou . La Pleine Lune a même été tenue pour responsable des transformations surnaturelles, transformant des hommes autrement inoffensifs en loups-garous féroces.

Célébration moderne de la pleine lune
La pleine lune inspire toujours les célébrations. L'un des rassemblements les plus célèbres de nos jours est la fête mensuelle de la pleine lune sur la plage de Haad Rin sur l'île de Koh Pha Ngan, en Thaïlande, où des milliers de touristes se réunissent chaque mois.